Open-loop steering articulated
This example simulates an articulated vehicle with an open loop sinusoidal steering input.
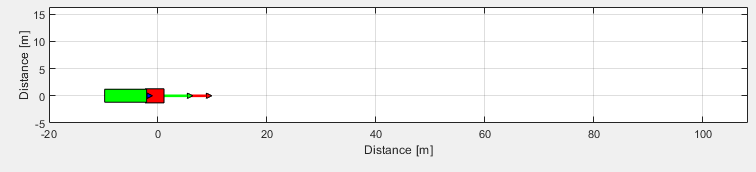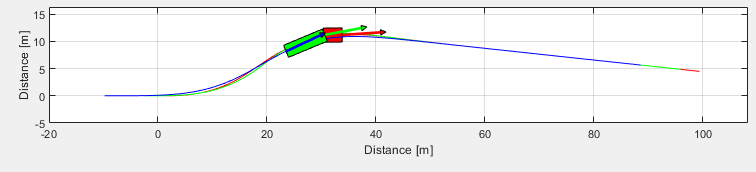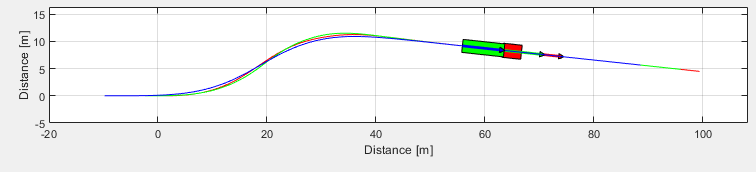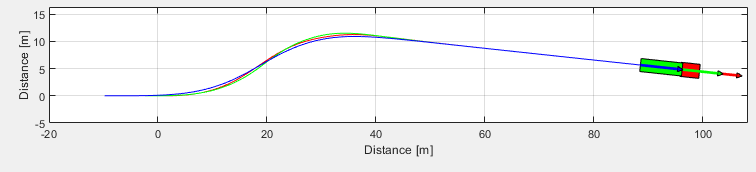
Contents
Simulation models and parameters
First, all classes of the package are imported with
clear ; close all ; clc import VehicleDynamicsLateral.*
Tire and vehicle model
Choosing tire and vehicle model. In this case, the parameters are defined by the user.
% Choosing tire TireModel = TirePacejka(); TireModel.a0 = 1; TireModel.a1 = 2; TireModel.a2 = 700; TireModel.a3 = 5000; TireModel.a4 = 80; TireModel.a5 = 0; TireModel.a6 = 0; TireModel.a7 = 0.6; % Choosing vehicle VehicleModel = VehicleArticulatedNonlinear(); VehicleModel.mF0 = 5200; VehicleModel.mR0 = 2400; VehicleModel.mF = 6000; VehicleModel.mR = 10000; VehicleModel.mM = 17000; VehicleModel.IT = 46000; VehicleModel.IS = 450000; VehicleModel.lT = 3.5; VehicleModel.lS = 7.7; VehicleModel.c = -0.3; VehicleModel.nF = 2; VehicleModel.nR = 4; VehicleModel.nM = 8; VehicleModel.wT = 2.6; VehicleModel.wS = 2.4; VehicleModel.muy = 0.8; VehicleModel.deltaf = 0; VehicleModel.Fxf = 0; VehicleModel.Fxr = 0; VehicleModel.Fxm = 0; % The System is completely defined once we atribute the chosen tire model % to the vehicle object. VehicleModel.tire = TireModel;
Simulation parameters
Choosing simulation time span
T = 10; % Total simulation time [s] resol = 80; % Resolution TSPAN = 0:T/resol:T; % Time span [s]
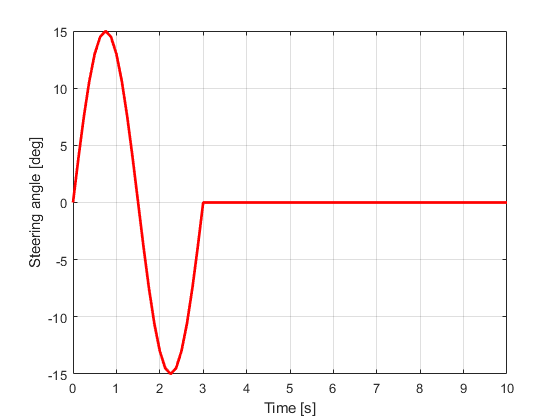
Open-loop steering input
Single period sine wave with:
delta_amplitude = 15*pi/180; % Steering input amplitude [rad] T_period = 3; % Steering single period [s] freq = 1/T_period; % Steering frequency [Hz] delta_freq = freq*2*pi; % Steering frequency [rad/s] % End input index sine_index = find(TSPAN == T_period); % Defining steering input VehicleModel.deltaf = [delta_amplitude*sin(delta_freq*TSPAN(1:sine_index)) zeros(1,length(TSPAN)-sine_index)]; figure hold on ; grid on ; box on plot(TSPAN,VehicleModel.deltaf*180/pi,'r','linewidth',2) xlabel('Time [s]') ylabel('Steering angle [deg]')
Run simulation
To define a simulation object (simulator) the arguments must be the vehicle object and the time span.
simulator = Simulator(VehicleModel, TSPAN);
Initial conditions Changing initial conditions of the simulation object
simulator.V0 = 40/3.6; % Initial velocity [m/s]
To simulate the system we run the Simulate method of the simulation object.
simulator.Simulate();
Results
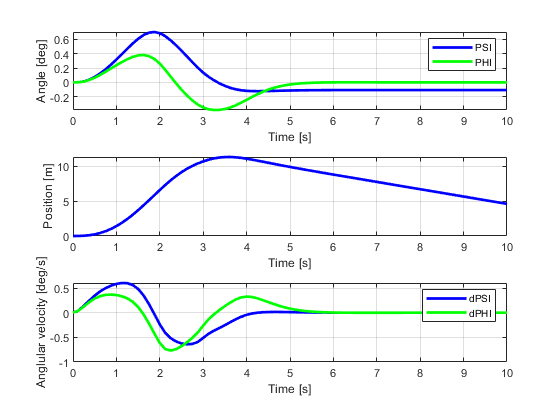
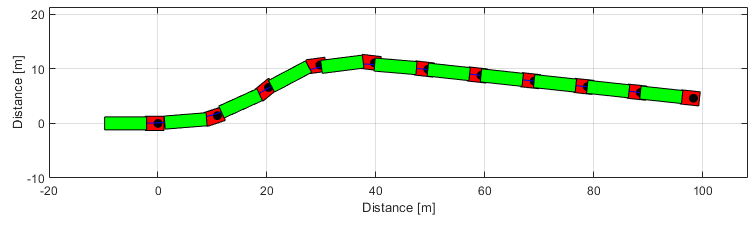
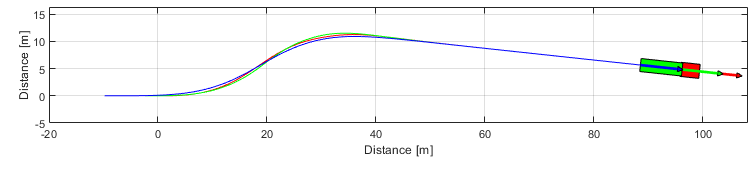
% Retrieving states YT = simulator.YT; PSI = simulator.PSI; PHI = simulator.PHI; dPSI = simulator.dPSI; dPHI = simulator.dPHI; figure subplot(3,1,1) hold on ; grid on ; box on plot(TSPAN,PSI,'b','linewidth',2) plot(TSPAN,PHI,'g','linewidth',2) ylabel('Angle [deg]') xlabel('Time [s]') legend('PSI','PHI') subplot(3,1,2) hold on ; grid on ; box on plot(TSPAN,YT,'b','linewidth',2) ylabel('Position [m]') xlabel('Time [s]') subplot(3,1,3) hold on ; grid on ; box on plot(TSPAN,dPSI,'b','linewidth',2) plot(TSPAN,dPHI,'g','linewidth',2) ylabel('Anglular velocity [deg/s]') xlabel('Time [s]') legend('dPSI','dPHI') g = Graphics(simulator); g.TractorColor = 'r'; g.SemitrailerColor = 'g'; g.Frame(); g.Animation(); % g.Animation('html/OpenLoopSteeringArticulated'); % Uncomment to save animation gif